The constants 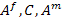 and and 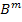 can be determined in terms of can be determined in terms of 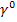 by solving Equations (7.244), (7.247), the first of Equations(7.248) and (7.249). These are by solving Equations (7.244), (7.247), the first of Equations(7.248) and (7.249). These are
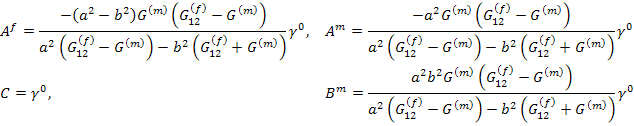 |
(7.250) |
At last, at the outer boundary the shear stress 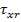 must match the shear stress must match the shear stress 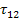 in coordinate system. Thus, at in coordinate system. Thus, at 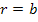 , the shear stress then becomes , the shear stress then becomes
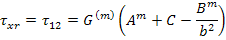 |
(7.251) |
The right hand side of above equation can be written as
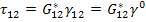 |
(7.252) |
Thus, the equivalent axial shear modulus can be given combining Equation (7.251) and Equation (7.252). Then values of constants 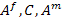 and and 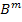 are substituted from Equation (7.250). Thus, we get the result are substituted from Equation (7.250). Thus, we get the result
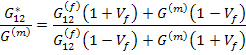 |
(7.253) |
|