|
Coefficient of mutual influence of first kind 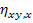 : :
Let us define the coefficient of mutual influence for the loading in Equation (5.60) as
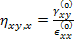 |
(5.67) |
For the loading of Equation (5.60) we have
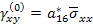 |
(5.68) |
Thus
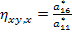 |
(5.69) |
Effective in-plane transverse modulus 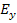 : :
The state of stress applied is
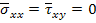 and and 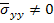 |
(5.70) |
For this state of stress, we get the relation as
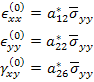 |
(5.71) |
Let us define the effective in-plane transverse modulus as
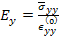 |
(5.72) |
This can be written as using the second of Equation (5.71) as
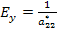 |
(5.73) |
|